Finding Slope On A Graph

It was learned before in Lesson 3 that the slope of the line on a position versus time graph is equal to the velocity of the object. If the object is moving with a velocity of +4 yard/s, then the slope of the line will be +4 g/s. If the object is moving with a velocity of -viii m/southward, then the slope of the line will exist -8 m/s. If the object has a velocity of 0 thou/south, then the slope of the line volition be 0 thou/s. The gradient of the line on a position versus time graph tells it all. Considering of its importance, a pupil of physics must have a good understanding of how to calculate the slope of a line. In this part of the lesson, the method for determining the gradient of a line on a position-time graph volition exist discussed.

Let's begin by considering the position versus fourth dimension graph beneath.

The line is sloping upwards to the right. Simply mathematically, past how much does it gradient upwards for every 1 2d along the horizontal (fourth dimension) axis? To answer this question we must use the gradient equation.

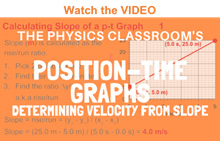
Using the Gradient Equation

The slope equation says that the gradient of a line is found by determining the amount of rise of the line between whatever two points divided by the corporeality of run of the line betwixt the same two points. In other words,

Choice ii points on the line and determine their coordinates.
Determine the difference in y-coordinates of these two points (ascension).
Decide the difference in 10-coordinates for these two points (run).
Separate the difference in y-coordinates by the departure in x-coordinates (rise/run or slope).

The diagram below shows this method being applied to decide the slope of the line. Note that three different calculations are performed for 3 different sets of ii points on the line. In each case, the result is the same: the slope is 10 m/south.

And then that was piece of cake - ascension over run is all that is involved.

Now allow'southward attempt a more difficult case. Consider the graph below. Notation that the gradient is not positive but rather negative; that is, the line slopes in the downward direction. Note besides that the line on the graph does not pass through the origin. Slope calculations are relatively easy when the line passes through the origin since 1 of the points is (0,0). But that is not the instance here. Test your understanding of gradient calculations by determining the slope of the line below. Then click the button to check your answer.

Cheque Your Agreement

1. Determine the velocity (i.east., slope) of the object as portrayed past the graph below. When you believe y'all know the respond (and not before), click the push button to check information technology.